81. If x is the distance from leading edge, then the boundary layer thickness in laminar flow varies as
82. Stanton diagram is a log-log plot of friction factor against Reynolds number
83. The depth 4d below the free surface at which the point velocity is equal to the
average velocity of flow for a uniform laminar flow with a free surface, will be 0.577D
84. The boundary layer thickness in turbulent flow varies as
85. The distance y from pipe boundary, at which the point velocity is equal to
average velocity for turbulent flow, is 0.223 R
86. If a sphere of diameter 1 cm falls in castor oil of kinematic viscosity 10 stokes, with a terminal velocity of 1.5 cm/sec, the coefficient of drag on the sphere is
160
87. In case of an airfoil, the separation of flow occurs at the extreme rear of body
88. When an ideal fluid flows past a sphere total drag is zero
89. With the same cross-sectional area and immersed in same turbulent flow, the
largest total drag will be on a circular disc of plate held normal to flow
90. In which of the following the friction drag is generally larger than pressure drag? an airfoil
91. For hydro-dynamically smooth boundary, the friction coefficient for turbulent flow is dependent only on Reynolds number
92. The value of friction factor T for smooth pipes for Reynolds number 106 is
approximately equal to 0.01
93. For laminar flow in a pipe of circular cross-section, the Darcy's friction factor f
is inversely proportional to Reynolds number and indpendent of pipe wall roughness
94. Separation of flow occurs when the boundary layer comes to rest
95. The loss of energy due to sudden enlargement is given by
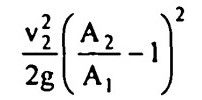
where A1, V1 are area of cross-section and velocity at entry and A2, v2, are area of
cross-section and velocity at exit.
96. The ratio of average velocity to maximum velocity for steady laminar flow in circular pipes is 1/2
97. The distance from pipe boundary, at which ,the turbulent shear stress' is one
third the wall shear stress, is 2/3 R
98. The discharge of a liquid of kinematic viscosity 4 cm²/sec through a 8 cm dia
meter pipe is 3200π cm³/sec. The type of flow expected is laminar flow
99. The Prandtl mixing length is zero at the pipe wall
100. The velocity distribution for laminar flow through a circular tube varies parabolically with maximum at the centre



Comments